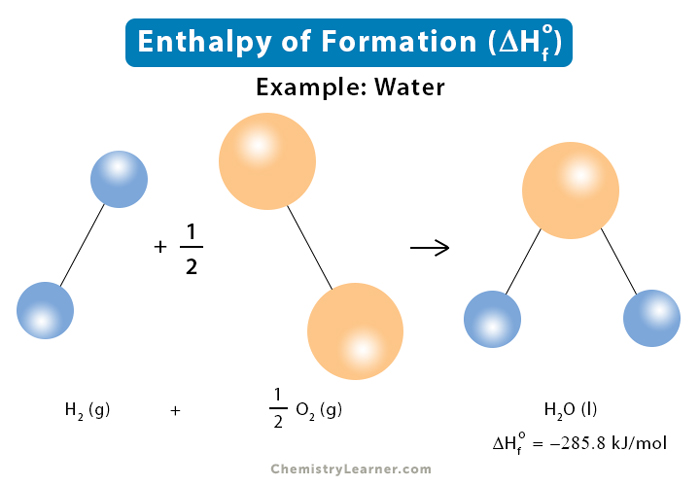
Standard Enthalpy of Formation
The standard enthalpy of formation, also known as the heat of formation, is the enthalpy change when 1 mole of a pure substance forms from its constituent elements at standard temperature and pressure. This quantity is handy for calculating or predicting enthalpy changes for chemical reactions that are unfeasible, unsafe to carry out, or challenging to measure [1-4].
The standard enthalpy of formation is denoted by the symbol ΔHfo. Its unit is kilojoule per mole or kJ/mol.
Examples [1-4]
1. Carbon Dioxide (CO2)
C (s, graphite) + O2 (g) → CO2 (g) ΔHfo = -393.509 kJ/mol
The standard enthalpy of the formation of carbon dioxide is -393.509 kJ/mol. It means that 393.509 KJ of energy is released when one mole of CO2 is formed from graphite (C) and oxygen gas (O2) at 1 atmospheric pressure and 25 ˚C. The reaction is exothermic.
2. Nitrogen Dioxide (NO2)
½ N2 (g) + O2 (g) → NO2 (g) ΔHfo = +33.2 kJ/mol
The standard enthalpy of the formation of nitrogen dioxide is +33.2 kJ/mol. It means that 33.2 kJ of energy is required to form one mole of NO2 from ½ mole of nitrogen (N2) and one mole of oxygen (O2) at 1 atmospheric pressure and 25 ˚C. The reaction is endothermic.
Below is a table of standard enthalpy of formation for many common substances [1].
| Compound | Standard Enthalpy of Formation, ΔHfo (kJ/mol) |
|---|---|
| O2 (g) | 0 |
| C (s, graphite) | 0 |
| CO (g) | – 110.5 |
| CO2 (g) | – 393.5 |
| H2 (g) | 0 |
| H2O (g) | – 241.8 |
| H2O (l) | – 285.8 |
| HF (g) | – 271.1 |
| NO (g) | 90.25 |
| NO2 (g) | 33.18 |
| N2O4 (g) | 9.16 |
| SO2 (g) | – 296.8 |
| SO3 (g) | – 395.7 |
| CH4 (g) | -74.81 |
| C2H5OH (l) | -275 |
| NH3 (g) | -46.1 |
| H2S (g) | -20.63 |
| NH4Cl (s) | -315.4 |
| NaCl (s) | -411.153 |
| MgO (s) | -601.7 |
Most values of standard enthalpy of formation are negative, indicating that these compounds are formed through exothermic reactions.
Characteristics of Enthalpy of Formation
1. The enthalpy of formation for any element in its standard form is assigned zero. The reason is that we do not make elements. We make compounds. Elements are found naturally on Earth. For example, carbon and oxygen are found in solid and gaseous forms, respectively, in nature. Therefore, ΔHfo {C(s)} = 0 and ΔHfo {O2(g)} = 0.
2. The enthalpy of formation will depend on the state of the product being formed. For example, the enthalpy of formation of gaseous water is higher than that of liquid water. The difference between them is the enthalpy of vaporization of water. Let us use the values of the two enthalpies to calculate the enthalpy of vaporization of water.
From the table,
ΔHfo {H2O(g)} = -241.8 kJ/mol
ΔHfo {H2O(l)} = -285.8 kJ/mol
The following formula gives the enthalpy of reaction.
ΔHrxn = ΣnΔHfo(products) – ΣmΔHfo(reactants)
In this case, the enthalpy of reaction is the enthalpy of vaporization. Therefore,
ΔHvap = (1 mol) ΔHfo {H2O(g)} – (1 mol) ΔHfo {H2O(l)} = (1 mol) (-241. 8 kJ/mol) – (1 mol) (-285.8 kJ/mol) = +44 kJ
H2O (l) → H2O (g) ΔHvap = +44 kJ/mol
44 kJ of energy is required to heat 1 mole of water at 1 atm pressure to convert it into vapor. As expected, this value comes out to be positive since heat is absorbed by water.
Solved Problems
Problem 1: Use standard enthalpies of formation to find the enthalpy of reaction for the following equation:
CH4 (g) + 2 O2 (g) → CO2 (g) + 2 H2O (l)
Solution
From the above table, the standard enthalpies of formation are as follows:
| Compound | Standard Enthalpy of Formation, ΔHfo (kJ/mol) |
| CH4 (g) | -74.81 |
| O2 (g) | 0 |
| CO2 (g) | -393.5 |
| H2O (l) | -285.8 |
The enthalpy of reaction is given by
ΔHrxn = ΣnΔHfo(products) – ΣmΔHfo(reactants)
Putting the values, we get
ΔHrxn = [(1 mol) ΔHfo {CO2 (g)} + (2 mol) ΔHfo {H2O(g)}] – [(1 mol) ΔHfo {CH4 (g)} + (2 mol) ΔHfo {O2(g)}]
=> ΔHrxn = [(1 mol) (-393.5 kJ/mol)] + (2 mol) (-285.8 kJ/mol)] – [(1 mol) (-74.81 kJ/mol) + (2 mol) (0)]
=> ΔHrxn = – 890.3 kJ/mol
Problem 2: Calculate ΔHrxno from the following reaction:
Mg (s) + 2 HCl (aq.) → H2 (g) + MgCl2 (aq.)
Use ΔHfo values from the table below.
| Compound | Standard Enthalpy of Formation, ΔHfo (kJ/mol) |
|---|---|
| HCl (g) | -92.3 |
| HCl (aq.) | -167.2 |
| MgCl2 (s) | -641.6 |
| MgCl2 (aq.) | -796.9 |
| H2O (l) | -285.8 |
Solution
The enthalpy of reaction is given by
ΔHrxn = ΣnΔHfo(products) – ΣmΔHfo(reactants)
Putting the values, we get
ΔHrxn= [(1 mol) ΔHfo {H2(g)} + (1 mol) ΔHfo {MgCl2(aq.)}] – [(1 mol) ΔHfo {Mg(s)} + (2 mol) ΔHfo {HCl(aq.)}]
=> ΔHrxn = [(1 mol) (0) + (1 mol) (-796.9 kJ/mol)] – [(1 mol) (0) + (2 mol) (-167.2 kJ/mol)]
=> ΔHrxn = -462.5 kJ/mol