Osmotic Pressure
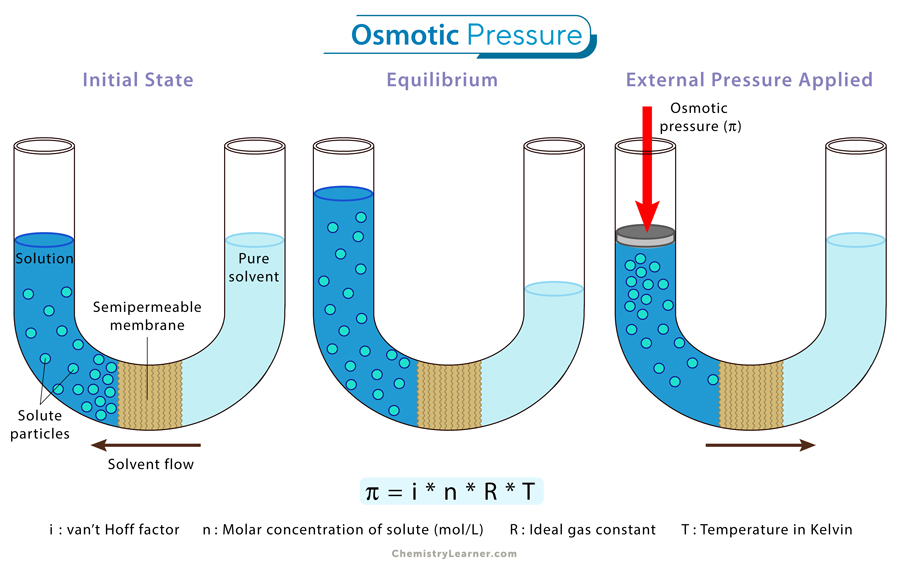
Osmotic pressure is the pressure required to prevent the flow of solvent through a semipermeable membrane from a dilute solution to a concentrated one. It is a colligative property, meaning it depends solely on the concentration of solute particles in the solution, regardless of their identity. [1-4]
Theory
A semipermeable membrane separates a solution and a solvent. This special membrane allows only solvent molecules to pass through while blocking larger solute molecules. As a result, solvent molecules naturally move from the solvent side to the solution side, increasing the solution’s volume. This process is called osmosis.
Osmotic pressure is the force needed to stop this natural flow of solvent molecules through the membrane. By applying pressure to the solution side, the movement of the solvent can be halted, effectively countering osmosis.
Formula
The osmotic pressure is represented by the Greek letter π and is calculated using the following formula: [1-4]
π = i * M * R * T
Where
– i: van’t Hoff factor
– M: molarity of the solution
– R: ideal gas constant
– T: absolute temperature (K)
The molarity is given by the number of moles (n) of the solute per liter (V) of the solution:
M = n/V
And the number of moles is the amount of solute in grams (msolute) divided by its molar mass (Msolute)
n = msolute/Msolute
Therefore, the molarity is:
M = msolute/(Msolute * V)
The expression for osmotic pressure becomes:
π = (i * msolute * R * T)/(Msolute * V)
This equation is often used to calculate the molar mass of the solute since other quantities can be easily determined experimentally.
Units and Dimension
Osmotic pressure is measured in units of atmosphere or atm. Other units include Pascal or Pa, pounds per inch or psi, and torr. The dimensional formula is [M1L-1T-2].
It is essential to note that this formula applies to ideal solutions in which the solute particles do not interact with each other and do not undergo chemical reactions.
Examples of Osmotic Pressure [1-4]
Plants
Plants rely on osmotic pressure to sustain their upright form. A well-hydrated plant absorbs water and expands its cells, which contain various salts. This cellular expansion increases pressure on the cell walls, enabling the plant to maintain its upright posture. In contrast, when the plant lacks an adequate water supply, its cells become hypertonic and shrink as water is lost. Consequently, the plant wilts and loses its sturdy, upright stance.
Blood Colloid
In the circulatory system, osmotic pressure is essential for maintaining the balance of fluids between blood and tissues. Blood contains proteins, such as albumin, that are too large to pass through the blood vessel walls. These proteins create a high solute concentration in the blood, leading to significant osmotic pressure. This osmotic pressure draws water back into the blood vessels from the surrounding tissues, preventing excessive fluid loss and edema.
Desalination
Reverse osmosis utilizes osmotic pressure to achieve desalination. It employs a higher pressure than the osmotic pressure to drive the movement of water across a semipermeable membrane while leaving the salt behind.
Hydrostatic Pressure vs. Osmotic Pressure
Hydrostatic pressure and osmotic pressure are two different forces that affect fluid movement. While osmotic pressure represents the pressure required to prevent solvent from flowing into a solution through a semipermeable membrane, hydrostatic pressure is the pressure exerted by water at rest or when it is not flowing in a confined space. The force of gravity causes this pressure. Therefore, the factors that affect hydrostatic pressure are density, depth of the water column, and acceleration due to gravity. For instance, the greater the water density or deeper the water column, the higher the hydrostatic pressure. [5]
Example Problems
Problem 1. A solution contains 0.2 moles of glucose dissolved in 1 liter of water. Calculate the osmotic pressure of the solution at 25 °C. (R = 0.0821 Lˑatm/molˑK).
Solution:
Given: n = 0.2 moles, V = 1 liter, R = 0.0821 Lˑatm/molˑK, T = 25 °C = 25 + 273 = 298 K
Since glucose does not disassociate, its van’t Hoff factor is 1, i.e., i = 1
The osmotic pressure is given by:
π = i * M * R * T
=> π = 1 * (0.2 mol/ 1 L) * (0.0821 Lˑatm/molˑK) * (298 K)
=> π = 4.89 atm
The osmotic pressure of the solution at 25 °C is 4.89 atm.
Problem 2. Calculate the osmotic pressure of 4.5 % solution of cane sugar (sucrose) at the temperature of 17° C. (M.W. of sucrose = 342.3 g/mol)
Solution:
Given: Concentration = 4.5 %, T = 17 °C = 17 + 273 = 290 K, M.W. = 342.3 g/mol
The solution contains 4.5 g of sucrose in 100 mL solution. Therefore, the molality is:
M = msolute/(Msolute * V)
=>M = 4.5/(342.3 * 100 x 10-3)
=> M = 0.131 mol/L
For sucrose, i = 1
The osmotic pressure is given by
π = i * M * R * T
=> π = 1 * 0.131 mol/L * 0.0821 Lˑatm/molˑK * 290 K
=> π = 3.12 atm
The osmotic pressure of the solution at 17 °C is 3.12 atm.
Problem 3. The osmotic pressure of a sodium chloride solution at 300 K is 60 atmospheres. What is the molar concentration of sodium chloride in this solution?
Solution:
Given: π = 60 atm, T = 300 K
The osmotic pressure equation is
π = i * M * R * T
=> M = π/(i * R * T)
NaCl dissociates into two ions: Na+ and Cl–. Therefore, its van’t Hoff factor is 2, i.e., i = 2
The molarity of NaCl is
M = (60 atm)/(2 * 0.0821 Lˑatm/molˑK * 300 K)
=> M = 1.21 mol/L
The molar concentration of sodium chloride (NaCl) in the solution is 1.21 mol/L.
Problem 4. How much glucose per liter should be used for an intravenous solution to match the 7.65 atm at 37 °C osmotic pressure of blood? (M.W. of glucose = 180 g/mol)
Solution:
Given: π = 7.65 atm, T = 37 °C = 37 + 273 = 310 K, M.W. = 180 g/mol
For glucose, i = 1
The molar concentration of glucose is
M = π/(i * R * T)
=> M = 7.65 atm/(1 x 0.0821 Lˑatm/molˑK x 310 K)
=> M = 0.3 mol/L
The amount of glucose is:
mglucose = 0.3 mol x 180 g/mol = 54.1 g
An intravenous solution should contain 54.1 grams per liter of glucose to match the 7.65 atm at 37 °C osmotic pressure of blood.