Internal energy is the energy due to the random motion of atoms, molecules, or particles in a system. It is measured at the microscopic scale and includes all macroscopic energies that the system possesses ^[1-4], like:

• Kinetic energy due to translational, vibrational, and rotational motion
• Potential energy due to intermolecular forces

Internal Energy Examples

A glass of water sitting on a table has no apparent macroscopic potential or kinetic energy. However, it has internal energy because water molecules move randomly, with each molecule contributing to the overall internal energy of the water. Another example is a battery, which uses internal energy to produce chemical reactions ^[1,2].

Internal Energy Equation

The internal energy can vary irrespective of the molecules’ position or the shape of a container to which a liquid or gas is confined. The internal energy is a state function, not a path function. When a system goes from one state to another, the change in internal energy depends on the initial and final states and not on the process taken to make the change ^[3-6].

The symbol for internal energy is U and its unit is Joules or J. Typically, the internal energy is calculated per mole of a substance. For most practical purposes, the change in internal energy ΔU is usually calculated. Let us study the internal energy values for a few situations.

Case 1: To get the internal energy when a system goes from its initial state to its final state, we use the following equation.

ΔU = U_final – U_initial

Where

U_final : Internal energy of the final state

U_initial : Internal energy of the initial state

In a closed cycle, the system comes back to its initial state. Therefore, U_final = U_initial => ΔU = 0. Thus, the internal energy change is zero for a closed cycle.

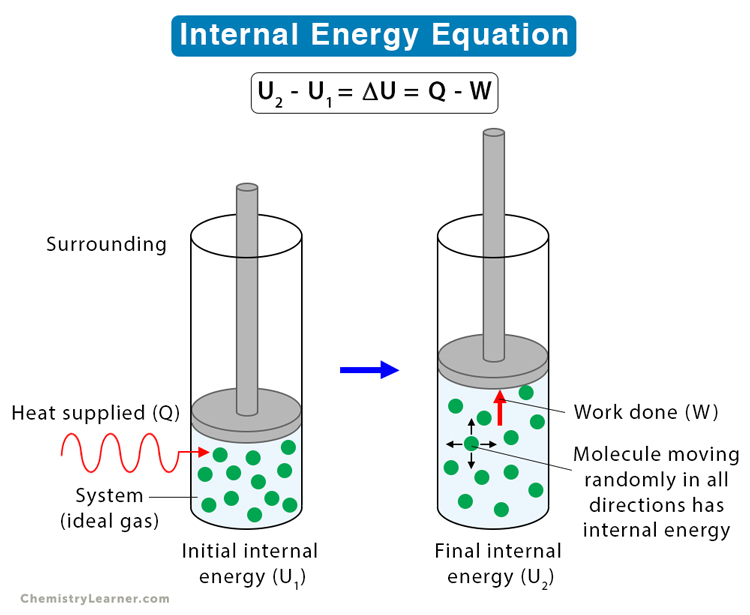
Case 2: Consider a system and its surrounding, as shown in the image below. The change in internal energy is related to heat supplied Q by the surrounding to the system and work done W by the system. From the first law of thermodynamics, this change is equal to the heat supplied minus the work done.

ΔU = Q – W

The work done can be mechanical, like the expansion of gases to move a piston. In such a case, suppose the gas applies pressure P on the piston, and its volume changes by ΔV. Then, the work done is

W = PΔV

And the internal energy is

ΔU = Q – PΔV

i. There is no heat exchange between the system and the surroundings for an adiabatic process. Hence, Q = 0, and the change of internal energy becomes

ΔU = – PΔV

ii. In an isochoric process, there is no change in volume or ΔV = 0. Therefore, the change in internal energy is

ΔU = Q

Case 3: An ideal gas is one whose molecules do not interact and obey the ideal gas law. Consider a monoatomic gas (ex. helium and neon) whose molecules exhibit translational motion only. The internal energy is given by

U = 3/2 nRT

Where,

n: Number of moles of the gas

R: Universal gas constant (R = 8.314 Jmol^-1K^-1)

T: Absolute temperature

One can see from the equation that the internal energy is a function of temperature and is directly proportional to it. The internal energy increases as the temperature increases, and vice versa. At absolute zero of zero Kelvin, the internal energy is zero. It means that the molecules stop moving and come to a standstill.

The change in internal energy of a monoatomic gas is given by

ΔU = 3/2 nRΔT

Thus, the change in internal energy is zero when there is no temperature change. For example, during an isothermal process.

When n = 1, we get the molar internal energy.

U_m = 3/2 RT

The internal energy per unit mass of the substance is the specific internal energy, denoted by the symbol u. It can be obtained by dividing the above equation by N_A, Avogadro’s number.

u = 3/2 (R/N_A) T

Or, u = 3/2 kT

Where k = R/N_A is the Boltzmann’s constant.

Internal Energy and Thermal Energy

We have seen that internal energy is the total of the potential and kinetic energy of the molecules in a substance. A part of internal energy that can be transferred from one substance to another is thermal energy. Thermal Energy is the heat content of a body and is present due to the virtue of temperature. Heat is the flow of thermal energy due to a temperature difference. It flows from a hot region (higher temperature) to a cold region (lower temperature) or from a hot body to a cold body. When heat is added to a substance, the particles start to vibrate and move more vigorously. Hence, the internal energy increases ^[7].