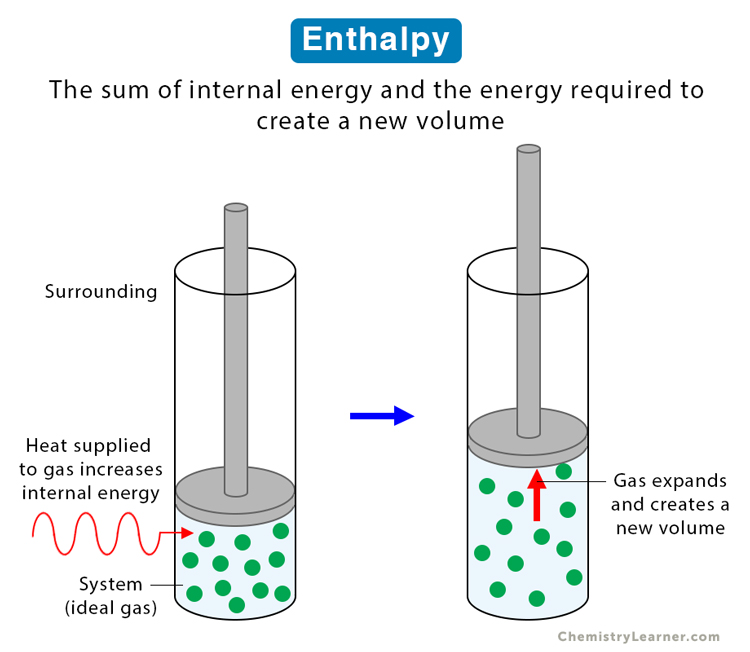
Enthalpy is a state function of a thermodynamic system and depends on other state functions. Mathematically, it is the sum of the internal energy and the product of the pressure and volume of the system. Enthalpy is the internal energy required to produce a system plus the energy necessary to make room for the system to expand. Expanding the system requires pressure to create a new volume and the product of the two results in work ^[1-4].

Suppose a system goes from one state to another through a thermodynamic process (physical or chemical). Since enthalpy is a state function, its change depends on the initial and final values of the internal energy, pressure, and volume and not on the process that caused the change. These three state variables are measurable quantities, thus making it easier to analyze enthalpy.

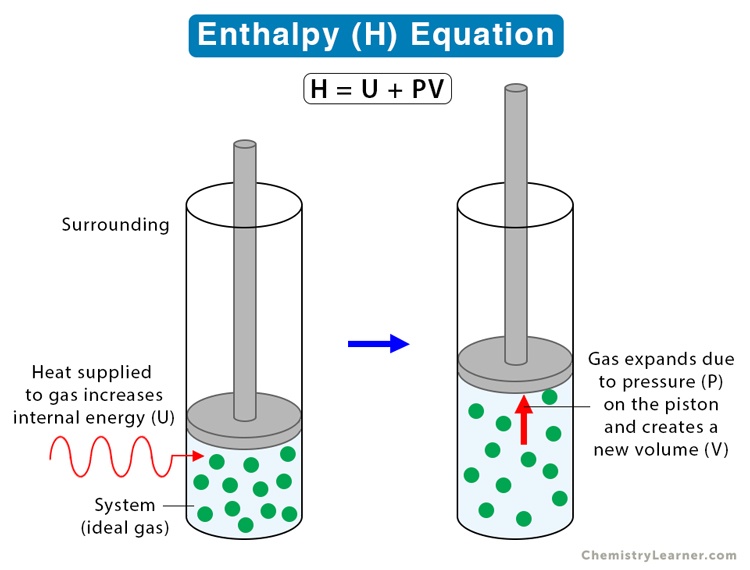
Enthalpy Equation

Suppose P, V, and U are the pressure, volume, and internal energy of a system. Then, the enthalpy H can be written in terms of P, V, and U as follows ^[1-8]:

H = U + PV

Where

H : Enthalpy

U : Internal Energy

P : Pressure

V : Volume

Symbol : H

SI Unit: Joule or J

Since it is an extensive property, the enthalpy is typically calculated for one mole of a substance. Hence, it is known as molar enthalpy, and its unit is Joule per mole or J/M. The enthalpy divided by the substance’s mass m gives the specific enthalpy, measured in Joules per kilogram or J/kg.

h = H/m

It is not so convenient nor necessary to determine the absolute values of enthalpy. To calculate the total enthalpy, one must know a reference point or zero point. However, this reference point is impossible to know. Any physical or chemical process involves a change in enthalpy, which can be measured experimentally.

Change in Enthalpy

From the first law of thermodynamics, we found that the change in internal energy ΔU is given by heat transfer Q between the system and the surroundings minus the work done W ^[1-8].

ΔU = Q – W

The heat and work terms depend on the process that causes the change. For an isobaric process resulting in expansion or contraction, the work done is given by the product of pressure P and change in volume ΔV.

W = PΔV

Therefore

ΔU = Q – PΔV

And the change in enthalpy is given by

ΔH = Q – PΔV + PΔV

Or, ΔH = Q

Thus, if a chemical or physical process occurs at constant pressure with the only work due to expansion or contraction, then the heat flow and enthalpy change for the process are equal. Chemists generally perform experiments under standard atmospheric conditions that occur at constant external pressure, making enthalpy the most suitable choice for estimating heat changes for chemical reactions.

Enthalpy from Heat Capacity

The heat flowing through a system can be written in terms of heat capacity at constant pressure C_P ^[2].

Q = C_PΔT

Or, ΔH = C_PΔT

Thus, the enthalpy change can also be considered the maximum amount of thermal energy obtainable from a thermodynamic process for which the pressure is kept constant. We divide the above equation by the mass of the system to obtain an expression for specific heat c_P.

Δh = c_PΔT

Or, c_p = (Δh/ΔT)_P

This expression gives the effect of temperature on enthalpy. It can be seen that the enthalpy increases with temperature. The enthalpy will increase when heat is added to a closed system at constant pressure.

Enthalpy for Phase Changes

We have found that the change in enthalpy is equal to the heat exchanged between the system and the surroundings. It is a valuable quantity as it tells us how much heat is required to melt or vaporize a substance at a constant temperature. In other words, there can be heat exchange at ΔT = 0. The heat Q is often called latent heat ^[2].

For example, if heat is supplied to change the phase of a substance from solid to liquid, it is known as latent heat or enthalpy of fusion. On the other hand, if heat is used to vaporize a substance, it is known as latent heat or enthalpy of vaporization. The heat of fusion and the heat of vaporization of water are given as follows.

ΔH_f = 6.01 kJM^-1 at 273.15 K

ΔH_v = 40.7 kJM^-1 at 373.15 K

Sign of Enthalpy Change

As mentioned before, the change of enthalpy can be used to study a thermodynamic process. The enthalpy change can be positive or negative. A negative value (ΔH < 0) indicates an exothermic process and a positive value (ΔH > 0) indicates an endothermic process. If the direction of the process is reversed, the sign of ΔH changes. This sign convention can be applied to a chemical reaction.

Example

When two moles of hydrogen (H₂) and one mole of oxygen (O₂) react to form water (H₂O), 572 kJ of energy is liberated. The enthalpy change is negative, and the reaction is exothermic.

2 H₂ (g) + O₂ (g) → 2 H₂O (l)    ΔH = -572 kJ

Enthalpy vs. Internal Energy

While enthalpy refers to the heat content, internal energy refers to the total energy. Both are state functions that are measured in Joules. The following table explains the difference between the two.

Parameter	Enthalpy	Internal Energy
Definition	Sum of internal energy and energy needed to create a physical environment	Sum of the kinetic and potential energy of all particles in a system
Symbol	H	U
Equation	H = U + PV	ΔU = Q – W or U = 3/2nRT (monoatomic gas)
Change	Change in enthalpy is negative for an exothermic process and positive for an endothermic process	Increases when the system absorbs energy and decreases when the system releases energy
Dependence	Internal energy, pressure, and volume	Temperature
When to Use	Suitable for an open system	Suitable for a closed system
Example	Ice absorbs heat and transforms into water with a change in enthalpy	A glass of water at a constant temperature has internal energy