First Order Reaction
What is a First-order Reaction
A first-order reaction is a chemical reaction where the reaction rate depends linearly on the reactant’s concentration. In other words, if the concentration is doubled, the reaction rate is also doubled. A first-order reaction can have one reactant, for example, a decomposition reaction, or two reactants [1-6].
First-order Reaction Rate Law
The kinetics of a chemical equation can be studied using rate law, which gives a relationship between the reaction rate and the concentration of the reactants. The rate law is expressed in differential and integral forms [1-7].
Differential Rate Law
The differential rate law gives the derivative of the reactant’s concentration with time. For a first-order reaction, it is given as,
R = – d[A]/dt = k [A]
Where,
R is the reaction rate
[A] is the concentration of the reactant A
k is the rate constant
The term d[A]/dt is the derivative of [A] with time.
Units
The unit for concentration is moles, the unit for the reaction rate is moles/second, and that for the rate constant is 1/second. Therefore, the numerical value of k for a first-order reaction is independent of the unit in which concentration is expressed.
Integrated Rate Law
The integrated rate law is beneficial in predicting how much of a reactant will remain at some time or how long it will take for the concentration to drop to some fixed amount (e.g., one-half). Integrating the above differential gives the expression for concentration as a function of time.
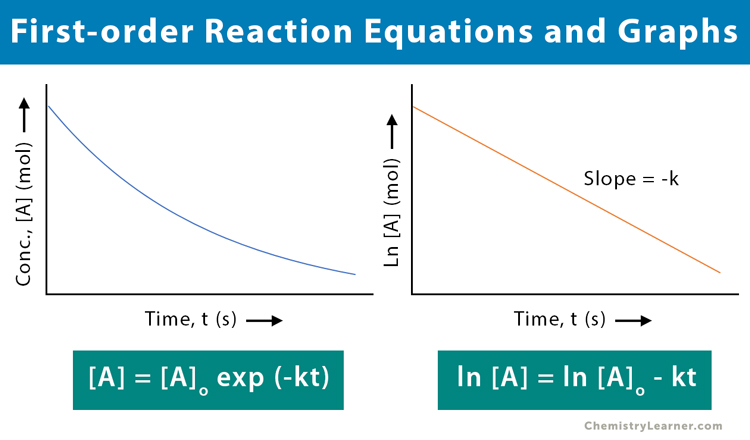
[A] = [A]o exp (-kt)
Where,
[A]o is the concentration at time t = 0
[A] is the concentration at time t
Taking the natural logarithm on both sides.
ln [A] = ln [A]o – kt
The above equation is a straight line with k as the slope. It can be used to determine the rate constant.
How to Determine the First-order Reaction
To determine if the reaction is first-order, plot the natural logarithm of the concentration versus time and see whether the graph is linear. The reaction will be first-order if the graph is linear with a negative slope.
Half-life of a First-order Reaction [9]
The half-life of a reaction is defined as the time taken for the reactant’s concentration to reduce to one-half.
[A] = [A]o / 2
Plugging in for [A] in the expression for [A], we get the half-life (t1/2).
t1/2 = 0.693 / k
Characteristics of First-order Reaction
Here are some facts and characteristics of the first-order reaction.
- The reaction rate is proportional to the reactant’s concentration
- Half-life is constant and independent of the reactant’s concentration
Examples of First-order Reaction
Here are some examples of first-order chemical reactions.
1. Trimethyl bromomethane ((CH3)3CBr) reacts with sodium hydroxide (NaOH) to give trimethyl methanol ((CH3)3COH) and sodium bromide (NaBr) [8].
(CH3)3CBr + NaOH → (CH3)3COH + NaBr
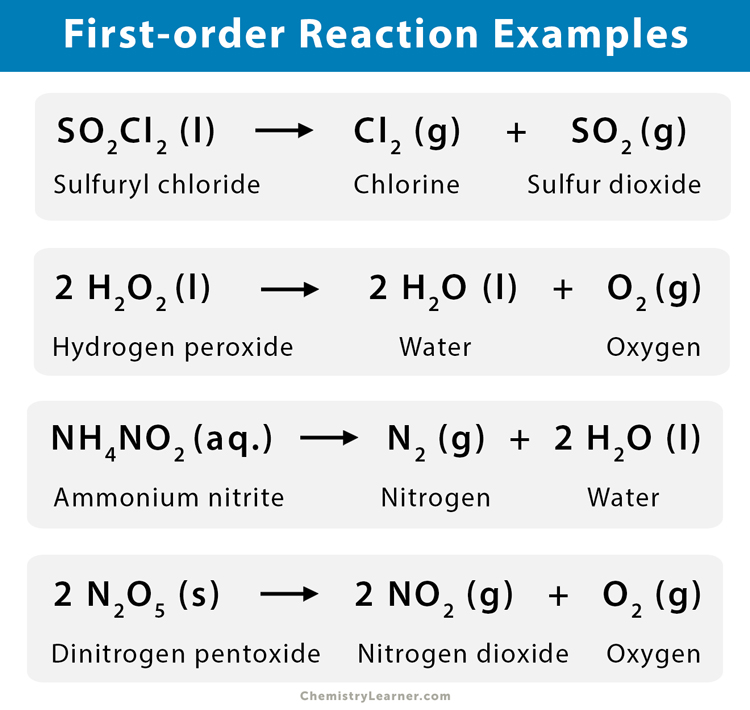
2. Decomposition of hydrogen peroxide (H2O2) into water (H2O) and oxygen (O2)
2 H2O2 (l) → H2O (l) + O2 (g)
3. Hydrolysis of methyl acetate (CH3COOCH3) in the presence of mineral acids
CH3COOCH3 (aq.) + H2O (l) → CH3COOH (aq. + CH3OH (aq.)
4. Decomposition of ammonium nitrite (NH4NO2) in aqueous solution
NH4NO2 (aq.) → N2 (g) + 2 H2O (l)
5. Hydrogenation of ethene (C2H4)
C2H4 (g) + H2 (g) → C2H6 (g)
Pseudo First-order Reaction
In some chemical reactions, the concentration of one of the reactants is so high that it does not change with time. In other words, the concentration remains constant throughout the reaction.
Consider the following reaction,
A + B → Products
The rate is given by,
R = k [A] [B]
This equation is the rate law for a second-order reaction. Suppose the concentration of B remains constant throughout the reaction. Then, it can be incorporated into the rate constant term k, and the rate R is given by,
R = k’ [A]
Where,
k’ = k [B] is the new rate constant.
Therefore, the pseudo first-order reaction is a second-order reaction that behaves like a first-order reaction.
Example: Hydrolysis of sucrose (C12H22O11) gives glucose (C6H12O6) and fructose (C6H12O6).
C12H22O11 + H2O → C6H12O6 + C6H12O6
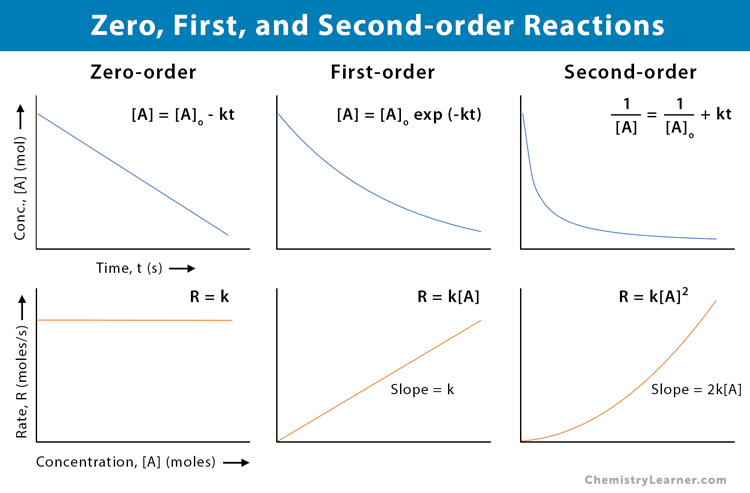
Zero, First, and Second-order Reactions
The following table lists the differences between the zero, first, and second-order reactions [10].
| Zero Order | First Order | Second Order | |
|---|---|---|---|
| Definition | The reaction rate is constant over time and independent of the reactants’ concentration | The reaction rate is proportional to the first power of the reactant’s concentration | The reaction rate is proportional to the second power of the reactant’s concentration or product of two reactants’ concentration |
| Rate equation | R = constant | R = k [A] | R = k [A]2 or R = k [A] [B] |
| Concentration | [A] = kt | [A] = [A]o exp (-kt) | 1 / [A] = 1 / [A]o + kt |
| Half-life | [A]o / 2k | 0.693 / k | 1 / k[A]o |
| Example | Decomposition of nitrous oxide | Decomposition of hydrogen peroxide | Decomposition of nitrogen dioxide |