Spin Quantum Number
What is the Spin Quantum Number
An electron orbiting around the nucleus has an intrinsic spin whose orientation is represented by the spin quantum number. Its value can determine the spin angular momentum along a specific axis using quantum theory [1-4].
According to Pauli Exclusion Principle, no two electrons can have the same set of quantum numbers. The electrons in an atom reside in orbitals determined by the magnetic quantum number. Hund’s Rule states that the maximum occupancy of electrons in each orbital is two. Hence, the two electrons will have the same values of the principal, azimuthal, and magnetic quantum numbers. They differ in their spin quantum number. It means that each electron has a unique spin.
Who Discovered Spin Quantum Number
Dutch-American physicists Samuel Goldsmith and George Uhlenbeck proposed the concept of electron spin in 1925.
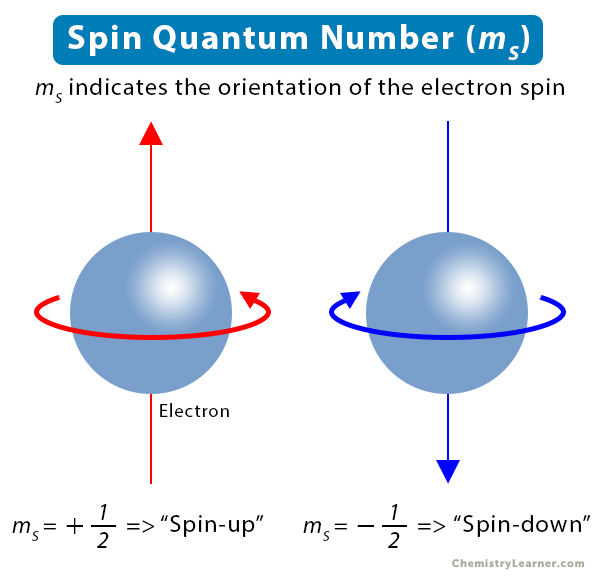
What are the Values of the Spin Quantum Number
The spin quantum number is denoted by the symbol ms. The only possible values of ms are +1/2 and -1/2. When ms = +1/2, the electron is in “spin-up” state. When ms = -1/2, the electron is in “spin-down” state. Thus, the two electrons occupying the same orbital have opposite spins and are paired [1-6].
How is Spin Quantum Number Applied to Atoms
The spin quantum number is the last among the four quantum numbers. The three other quantum numbers, principal quantum number (n), azimuthal quantum number (l), and magnetic quantum number (ml), decide the electron’s orbital. The spin quantum number determines the electron’s spin. Let us look at a few examples of atoms to illustrate this concept [5].
1. Hydrogen
Hydrogen has one electron which is in “spin-up” state. Therefore, it’s four quantum numbers are:
n = 1, l = 0, ml = 0, ms = +1/2
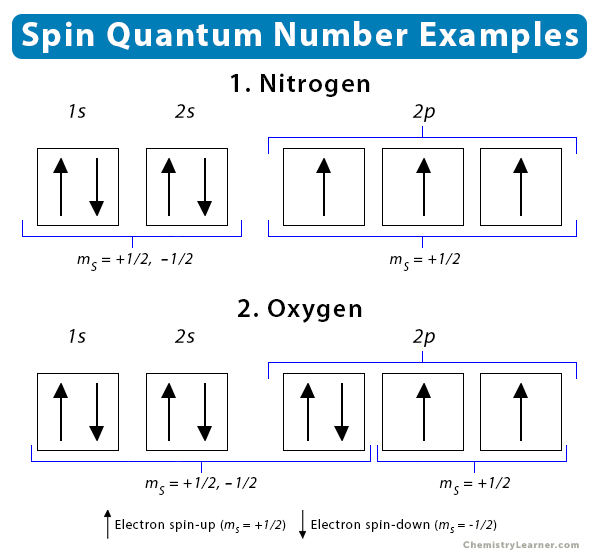
2. Nitrogen
The atomic number of nitrogen is 7, and its electron configuration is 1s2 2s2 2p3. Therefore, the four quantum numbers of its seven electrons are as follows:
| Subshell | Principal Quantum Number (n) | Azimuthal Quantum Number (l) | Magnetic Quantum Number (ml) | Spin Quantum Number (ms) |
|---|---|---|---|---|
| 1s | 1 | 0 | 0 | +1/2 |
| 1s | 1 | 0 | 0 | -1/2 |
| 2s | 2 | 0 | 0 | +1/2 |
| 2s | 2 | 0 | 0 | -1/2 |
| 2p | 2 | 1 | -1 | +1/2 |
| 2p | 2 | 1 | 0 | +1/2 |
| 2p | 2 | 1 | +1 | +1/2 |
Note that the three electrons in the 2p subshell have ms = +1/2. It means that they are “spin-up” and unpaired. This observation follows Hund’s Rule.
3. Oxygen
The atomic number of oxygen is 8, and its electron configuration is 1s2 2s2 2p4. The values of the four quantum numbers are listed in the table below.
| Subshell | Principal Quantum Number (n) | Azimuthal Quantum Number (l) | Magnetic Quantum Number (ml) | Spin Quantum Number (ms) |
|---|---|---|---|---|
| 1s | 1 | 0 | 0 | +1/2 |
| 1s | 1 | 0 | 0 | -1/2 |
| 2s | 2 | 0 | 0 | +1/2 |
| 2s | 2 | 0 | 0 | -1/2 |
| 2p | 2 | 1 | -1 | +1/2 |
| 2p | 2 | 1 | -1 | -1/2 |
| 2p | 2 | 1 | 0 | +1/2 |
| 2p | 2 | 1 | +1 | +1/2 |
The spin quantum number values of the electrons in nitrogen and oxygen are shown below.
Quantization of the Spin Angular Momentum
Like the orbital angular momentum, the spin angular momentum is also quantized and given by the following relationship [6].
S = √{s(s+1)} h/2π
Where,
S: spin angular momentum
s: spin quantum number
h: Planck’s constant (= 6.626 x 10-34 J.s)


Very nice