The Van der Waals equation is a modification of the ideal gas equation that accounts for the volume occupied by gas molecules and the attractive forces between them. While the ideal gas equation assumes that gas molecules have no volume and do not interact with each other, the Van der Waals equation considers these factors to provide a more accurate description of real gases. ^{[1 – 4]}

The Van der Waals equation adjusts for deviations from ideal behavior by incorporating correction factors for molecular size (volume) and intermolecular forces (attraction). It allows for a more precise prediction of gas behavior under conditions where these deviations become significant.

The equation is named after Dutch theoretical physicist and thermodynamicist Johannes Diderik van der Waals, who included it in his 1873 doctoral thesis. It takes the following form:

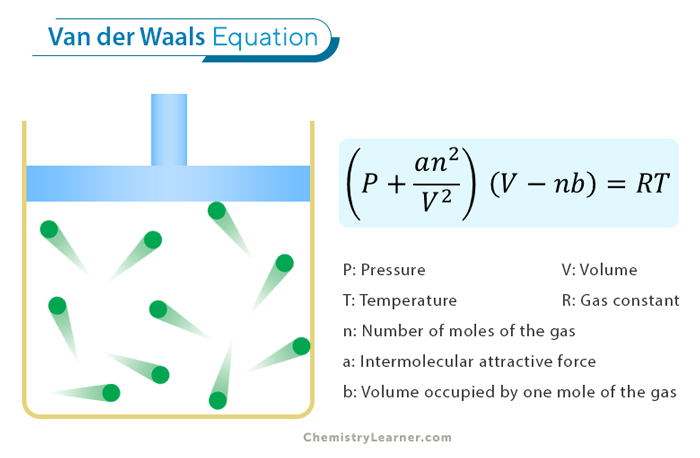
(P + an²/V²) (V – nb) = nRT

Where:

• P is the pressure.
• V is the volume.
• T is the temperature.
• R is the gas constant.
• a represents the strength of intermolecular attractive forces.
• b represents the volume occupied by one mole of the gas molecules.
• n is the number of moles of the gas.

Derivation of the Van der Waals Equation

The derivation of the Van der Waals equation involves modifying the ideal gas law, PV = nRT, by incorporating correction terms for molecular size and intermolecular interactions. We begin by considering a real gas whose key assumptions are that gas molecules have finite volumes and experience attractive forces that reduce their pressure and increase their volume. ^[1]

Pressure Correction

In the Van der Waals equation, the pressure correction factor ‘a’ accounts for the attractive forces between gas molecules, which is not considered in the ideal gas law. This correction factor represents the strength of these forces and adjusts for the deviation of real gases from ideal behavior. The corrected pressure term is then P + an²/V², reflecting the increase in pressure due to attractive forces. The unit of a is L²⋅atm/mol².

Volume Correction

The Van der Waals volume correction factor, denoted as ‘b’, corrects the molecular volume in this equation. When using the Van der Waals equation, it is essential to include the correction factor ‘b’ to adjust for the space occupied by the gas molecules. It helps provide a more accurate representation of real gases, especially at higher pressures and lower temperatures where intermolecular forces become significant. Therefore, the corrected volume term becomes V – nb. The unit of b is L/mol.

Merits

When describing real gas behavior, the Van der Waals equation offers significant advantages over the Ideal Gas Law. Unlike the Ideal Gas Law, which assumes ideal conditions of no volume and intermolecular forces, the Van der Waals equation accounts for the finite volume of gas particles and their attractive forces. ^[1]

By incorporating corrections for particle volume and intermolecular interactions, the Van der Waals equation provides a more accurate description of gas behavior, particularly at high pressures and low temperatures where deviations from ideal behavior are pronounced. It is a valuable tool in various scientific and industrial applications requiring precise gas calculations.

Demerits and Limitations

While the Van der Waals equation is useful for approximating real gas behavior, it is important to know its limitations and assumptions. One key limitation is that the equation assumes gas particles have negligible volume and do not interact with each other. This simplification may not hold under certain conditions, such as at high pressures or low temperatures. ^[1]

Additionally, the Van der Waals equation may not be appropriate for gases deviating significantly from ideal behavior, such as polar or gases with strong intermolecular forces. In such cases, more complex equations of state or experimental data may be needed to describe the gas behavior accurately.

It is important to exercise caution when using the Van der Waals equation and consider its limitations before applying it when its assumptions may not hold.

Example Problems with Solutions

Problem 1: One mol of a gas occupies a volume of 2.5 L at a temperature of 300 K. The Van der Waals constants for this gas are a = 3.59 L²⋅atm/mol² and b = 0.0427 L/mol. Calculate the pressure of the gas using the Van der Waals equation.

Solution:

The Van der Waals equation is given by:

(P + an²/V²) (V – nb) = nRT

We are given:

V = 2.5 L, T = 300 K, a = 3.59 L²⋅atm/mol², b = 0.0427 L/mol, and n = 1. Also, R = 0.082 Lˑatm/molˑK

We need to rearrange the equation and then solve for P.

P = RT/(V-nb) – an²/V²

=> P = (0.082 Lˑatm/molˑK x 300 K)/(2.5 L – 1 mol x 0.0427 L/mol) – 3.59 L²⋅atmˑmol^-2 x 1 mol²/(2.5 L)²

=> P = 9.44 atm

Problem 2: Consider a sample of nitrogen gas​ at a temperature of 300 K and a pressure of 10 atm. The Van der Waals constants for nitrogen are a = 1.39 L²⋅atm/mol² and b = 0.0391 L/mol. Calculate the molar volume of the nitrogen gas using the Van der Waals equation.

The Van der Waals equation for one mole is given by:

(P + a/V²) (V – b) = RT

We are given:

P = 10 atm, T = 300 K, a = 1.39 L²⋅atm/mol², b = 0.0391 L/mol. Also, R = 0.082 Lˑatm/molˑK

We need to substitute these values, rearrange the equation, and solve for V.

(10 + 1.39/V²)(V – 0.0391) = 0.0821 ˑ 300

=>10 V² – 25.021 V + 1.346 = 0

=> V = 2.474 L/mol

Note that we have ignored the negative value of V since the volume cannot be negative.